Magnet Fields and What They Do

Roger Hinrichs; Paul Peter Urone; Paul Flowers; Edward J. Neth; William R. Robinson; Klaus Theopold; Richard Langley; Julianne Zedalis; John Eggebrecht; E.F. Redish

32 Magnet Fields and What They Do

Magnetic Fields and Magnetic Field Lines

Derived from Magnetic Fields and Magnetic Field Lines by OpenStax

Einstein is said to have been fascinated by a compass as a child, perhaps musing on how the needle felt a force without direct physical contact. His ability to think deeply and clearly about action at a distance, particularly for gravitational, electric, and magnetic forces, later enabled him to create his revolutionary theory of relativity. Since magnetic forces act at a distance, we define a magnetic field to represent magnetic forces. The pictorial representation of magnetic field lines is very useful in visualizing the strength and direction of the magnetic field. As shown in Figure 1, the direction of magnetic field lines is defined to be the direction in which the north end of a compass needle points. The magnetic field is traditionally called the B-field.

Instructor’s Note

Why do we use B for magnetic field? I have no idea.

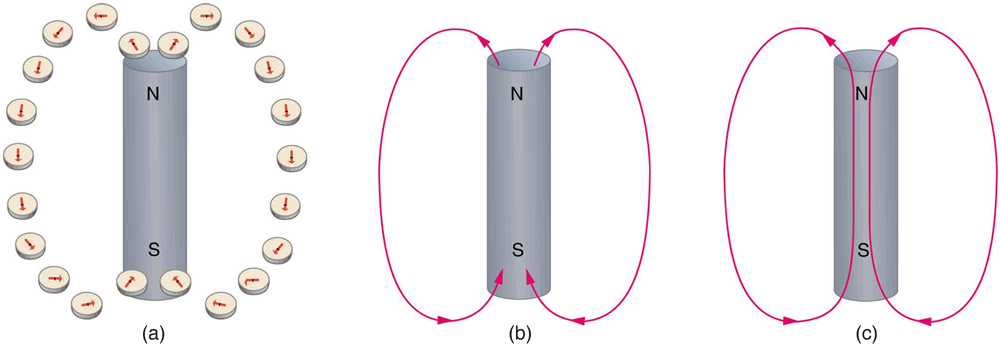
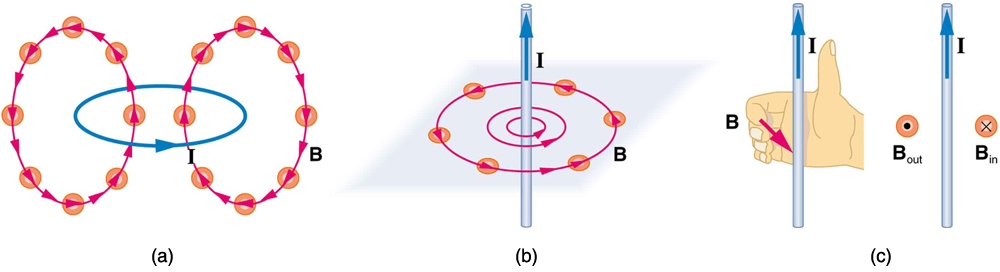
**Figure 1**. Magnetic field lines are defined to have the direction that a small compass points when placed at a location. (a) If small compasses are used to map the magnetic field around a bar magnet, they will point in the directions shown: away from the north pole of the magnet, toward the south pole of the magnet. (Recall that the Earth’s north magnetic pole is really a south pole in terms of definitions of poles on a bar magnet.) (b) Connecting the arrows gives continuous magnetic field lines. The strength of the field is proportional to the closeness (or density) of the lines. (c) If the interior of the magnet could be probed, the field lines would be found to form continuous closed loops.

Small compasses used to test a magnetic field will not disturb it. (This is analogous to the way we tested electric fields with a small test charge. In both cases, the fields represent only the object creating them and not the probe testing them.) Figure 2 shows how the magnetic field appears for a current loop and a long straight wire, as could be explored with small compasses. A small compass placed in these fields will align itself parallel to the field line at its location, with its north pole pointing in the direction of B. Note the symbols used for field into and out of the paper.

**Figure 2.** Small compasses could be used to map the fields shown here. (a) The magnetic field of a circular current loop is similar to that of a bar magnet. (b) A long and straight wire creates a field with magnetic field lines forming circular loops. (c) When the wire is in the plane of the paper, the field is perpendicular to the paper. Note that the symbols used for the field pointing inward (like the tail of an arrow) and the field pointing outward (like the tip of an arrow).

Making Connections: Concept of a Field

A field is a way of mapping forces surrounding any object that can act on another object at a distance without apparent physical connection. The field represents the object generating it. Gravitational fields map gravitational forces, electric fields map electrical forces, and magnetic fields map magnetic forces.

Extensive exploration of magnetic fields has revealed a number of hard-and-fast rules. We use magnetic field lines to represent the field. The properties of magnetic field lines can be summarized by these rules:

The direction of the magnetic field is tangent to the field line at any point in space. A small compass will point in the direction of the field line.
The strength of the field is proportional to the closeness of the lines. It is exactly proportional to the number of lines per unit area perpendicular to the lines (called the areal density).
Magnetic field lines can never cross, meaning that the field is unique at any point in space.
Magnetic field lines are continuous, forming closed loops without beginning or end. They go from the north pole to the south pole.

The last property is related to the fact that the north and south poles cannot be separated. It is a distinct difference from electric field lines, which begin and end on the positive and negative charges. If magnetic monopoles existed, then magnetic field lines would begin and end on them.

Homework

2. Compasses and magnetic field lines

Section Summary

Magnetic fields can be pictorially represented by magnetic field lines, the properties of which are as follows:
- The field is tangent to the magnetic field line.
- Field strength is proportional to the line density.
- Field lines cannot cross.
- Field lines are continuous loops.

Magnetic Field Strength: Force on a Moving Charge in a Magnetic Field

Derived from Magnetic Field Strength: Force on a Moving Charge in a Magnetic Field by OpenStax

Instructor’s Note

In this section I am looking for you to understand:

Only moving charges (i.e. currents) experience forces due to magnetic fields
The letters in the expression 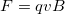$F = qvB$ . As far as prep is concerned, the angle theta will always be 90-degrees, so sine will always be 1.

What is the mechanism by which one magnet exerts a force on another? The answer is related to the fact that all magnetism is caused by current, the flow of charge. Magnetic fields exert forces on moving charges, and so they exert forces on other magnets, all of which have moving charges.

Right Hand Rule 1

The magnetic force on a moving charge is one of the most fundamental known. Magnetic force is as important as the electrostatic or Coulomb force. Yet the magnetic force is more complex, in both the number of factors that affects it and in its direction, than the relatively simple Coulomb force. The magnitude of the magnetic force $F$ on a charge $q$ moving at a speed $v$ in a magnetic field of strength $B$ is given by

$F = qvB \sin{\theta}$

Instructor’s Note

The only thing about the angle you need to know is that if the velocity if PARALLEL to the field, then there is no force!

where $\theta$ is the angle between the directions of $v$ and $B$ . This force is often called the Lorentz force. In fact, this is how we define the magnetic field strength $B$ —in terms of the force on a charged particle moving in a magnetic field. The SI unit for magnetic field strength $B$ is called the tesla (T) after the eccentric but brilliant inventor Nikola Tesla (1856–1943). To determine how the tesla relates to other SI units, we solve $F = qvB \sin{\theta}$ for $B$ .

$B = \frac{F}{qv \sin{\theta}}$

Instructor’s Note

We will spend time in class thinking about these complex directions!

Because $\sin{\theta}$ is unitless, the tesla is

$1 \text{ T} = \frac{1 \text{ N}}{\text{C } \cdot \text{ m/s}} = \frac{1 \text{ N}}{\text{A } \cdot \text{ m}}$

(note that C/s = A).

Another smaller unit, called the gauss (G), where $1 \text{ G} = 10^{-4} \text{ T}$ , is sometimes used. The strongest permanent magnets have fields near 2 T; superconducting electromagnets may attain 10 T or more. The Earth’s magnetic field on its surface is only about $5 \text{ } \times \text{ } 10^{-5} \text{ T}$ , or 0.5 G.

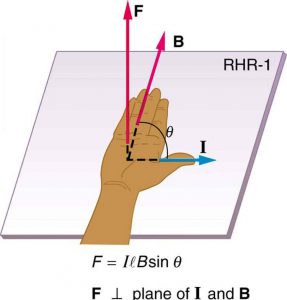
The direction of the magnetic force $F$ is perpendicular to the plane formed by $v$ and $B$ , as determined by the right hand rule 1 (or RHR-1), which is illustrated in Figure 1. RHR-1 states that, to determine the direction of the magnetic force on a positive moving charge, you point the thumb of the right hand in the direction of $v$ , the fingers in the direction of $B$ , and a perpendicular to the palm points in the direction of $F$ . One way to remember this is that there is one velocity, and so the thumb represents it. There are many field lines, and so the fingers represent them. The force is in the direction you would push with your palm. The force on a negative charge is in exactly the opposite direction to that on a positive charge.

Instructor’s Note

Again, we will spend more time in class going over these complex directions!

Magnetism is WAY more interesting then just bar magnets!

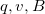**Figure 1.** Magnetic fields exert forces on moving charges. This force is one of the most basic known. The direction of the magnetic force on a moving charge is perpendicular to the plane formed by $v$ and $B$ and follows right hand rule–1 (RHR-1) as shown. The magnitude of the force is proportional to $q, v, B$ , and the sine of the angle between $v$ and $B$ .

Making Connections: Charges and Magnets

There is no magnetic force on static charges. However, there is a magnetic force on moving charges. When charges are stationary, their electric fields do not affect magnets. But, when charges move, they produce magnetic fields that exert forces on other magnets. When there is relative motion, a connection between electric and magnetic fields emerges—each affects the other.

Calculating Magnetic forces

Instructor’s Note

This is a good example as I will expect you to solve for the numbers in problems such as this on your homework and on your quiz. I will NOT ask you about the directions.

With the exception of compasses, you seldom see or personally experience forces due to the Earth’s small magnetic field. To illustrate this, suppose that in a physics lab you rub a glass rod with silk, placing a 20-nC positive charge on it. Calculate the force on the rod due to the Earth’s magnetic field $5.0 \times 10^{-5} \text{ T}$ , if you throw it with a horizontal velocity of 10 m/s due west in a place where the Earth’s field is due north parallel to the ground. (The direction of the force is determined with right hand rule 1 as shown in Figure 2.)

**Figure 2.** A positively charged object moving due west in a region where the Earth’s magnetic field is due north experiences a force that is straight down as shown. A negative charge moving in the same direction would feel a force straight up.

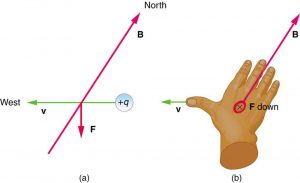
Strategy

We are given the charge, its velocity, and the magnetic field strength and direction. We can thus use the equation $F = qvB \sin{\theta}$ to find the force.

Solution

The magnetic force is

$F = qvB \sin{\theta}$

We see that $\sin{\theta} = 1$ , since the angle between the velocity and the direction of the field is $90º$ . Entering the other given quantities yields

$F = (20 \times 10^{-9} \text{ C})(10 \text{ m/s})(5 \times 10^{-5} \text{ T})$

$= 1 \times 10^{-11} (\text{C } \cdot \text{ m/s})(\frac{\text{N}}{\text{C} \cdot \text{m/s}}) = 1 \times 10^{-11} \text{ N}$ .

Discussion

This force is completely negligible on any macroscopic object, consistent with experience. (It is calculated to only one digit, since the Earth’s field varies with location and is given to only one digit.) The Earth’s magnetic field, however, does produce very important effects, particularly on submicroscopic particles. Some of these are explored in Force on a Moving Charge in a Magnetic Field: Examples and Applications.

Section Summary

Magnetic fields exert a force on a moving charge q, the magnitude of which is

$F = qvB \sin{\theta}$

where $\theta$ is the angle between the directions of $v$ and $B$ .

The SI unit for magnetic field strength $B$ is the tesla (T), which is related to other units by

$1 \text{ T} = \frac{1 \text{ N}}{\text{C } \cdot \text{ m/s}} = \frac{1 \text{ N}}{\text{A } \cdot \text{ m}}$

The direction of the force on a moving charge is given by right hand rule 1 (RHR-1): Point the thumb of the right hand in the direction of $v$ , the fingers in the direction of $B$ , and a perpendicular to the palm points in the direction of $F$ .
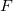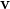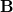The force is perpendicular to the plane formed by $\textbf{v}$ and $\textbf{B}$ . Since the force is zero if 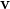$\textbf{v}$ is parallel to 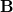$\textbf{B}$ , charged particles often follow magnetic field lines rather than cross them.

Homework

3. Units of magnetic field.

4. What will feel a magnetic force.

5. What will feel a magnetic force – macroscopic objects.

6. Thinking about the magnetic forces on charged particles.

7. Magnetic force on an airplane.

8. Magnetic force on a baseball.

9. Magnetic force on an electron at an angle to $\vec{B}$ .

Magnetic Force on a Current-Carrying Conductor

Derived from Magnetic Force on a Current-Carrying Conductor by OpenStax

Instructor’s Note

As with the section on magnetic forces on moving charged particles, I am NOT expecting you to master all of the material in this section. I am hoping that you will, by the end of this section:

Know that only currents perpendicular to magnetic fields experience magnetic forces
Be able to calculate the magnetic force on a section of wire of length L carrying a current I perpendicular to a magnetic field B using F=ILB

We will, as with charged particles, deal with the directions and the case of currents neither parallel or perpendicular to magnetic fields in class.

Because charges ordinarily cannot escape a conductor, the magnetic force on charges moving in a conductor is transmitted to the conductor itself.

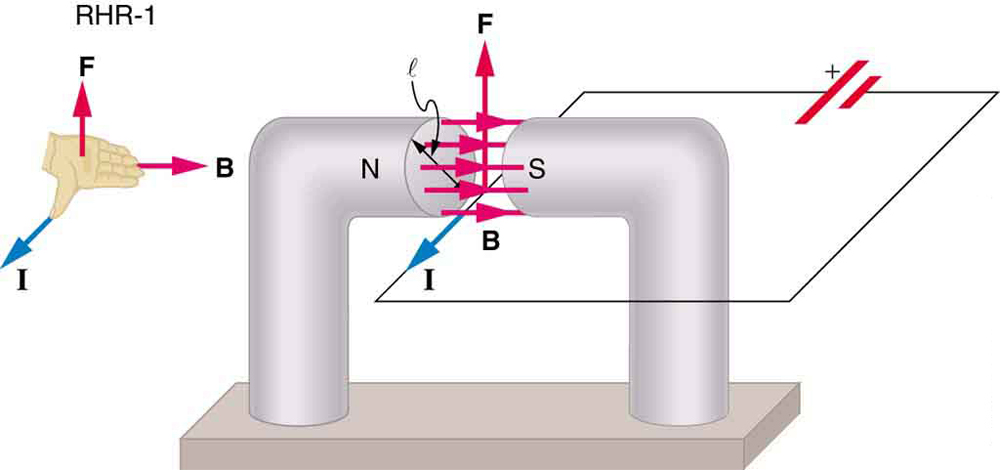
**Figure 1.** The magnetic field exerts a force on a current-carrying wire in a direction given by the right hand rule 1 (the same direction as that on the individual moving charges). This force can easily be large enough to move the wire, since typical currents consist of very large numbers of moving charges.

We can derive an expression for the magnetic force on a current by taking a sum of the magnetic forces on individual charges. (The forces add because they are in the same direction.) The force on an individual charge moving at the drift velocity $v_d$ is given by $F = qv_{d}B \sin{\theta}$ . Taking $B$ to be uniform over a length of wire $l$ and zero elsewhere, the total magnetic force on the wire is then $F = (qv_{d}B \sin{\theta})(N)$ , where $N$ is the number of charge carriers in the section of wire of length $l$ . Now, $N = nV$ , where $n$ is the number of charge carriers per unit volume and $V$ is the volume of wire in the field. Noting that $V = Al$ , where $A$ is the cross-sectional area of the wire, then the force on the wire is $F = (qv_{d}B \sin{\theta})(nAl)$ . Gathering terms,

$F = (nqAv_{d})lB \sin{\theta}$

Because $nqAv_{d} = I$ (see Current),

$F = IlB \sin{\theta}$

is the equation for magnetic force on a length $l$ of wire carrying a current $I$ in a uniform magnetic field $B$ , as shown in Figure 2. If we divide both sides of this expression by $l$ , we find that the magnetic force per unit length of wire in a uniform field is $\frac{F}{l} = IB \sin{\theta}$ . The direction of this force is given by RHR-1, with the thumb in the direction of the current $I$ . Then, with the fingers in the direction of $B$ , a perpendicular to the palm points in the direction of $F$ , as in Figure 2.

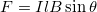**Figure 2.** The force on a current-carrying wire in a magnetic field is $F = I l B \sin{\theta}$ . Its direction is given by RHR-1.

Calculating Magnetic Force on a Current-Carrying Wire: A Strong Magnetic Field

Calculate the force on the wire shown in Figure 1, given $B = 1.50 \text{ T}, l = 5.00 \text{ cm}$ and $I = 20.0 \text{ A}$ .

Strategy

The force can be found with the given information by using $F = I l B \sin{\theta}$ and noting that the angle $\theta$ between $I$ and $B$ is 90º, so that $\sin{\theta}$ .

Solution

Entering the given values into $F = I l B \sin{\theta}$ yields

$F = I l B \sin{\theta} = (20.0 \text{ A})(0.0500 \text{ m})(1.50 \text{ T})(1)$ .

The units for tesla are $1 \text{ T} = \frac{N}{\text{A} \cdot \text{m}}$ ; thus,

$F = 1.50 \text{ N}$ .

Discussion

This large magnetic field creates a significant force on a small length of wire.

Magnetic force on current-carrying conductors is used to convert electric energy to work. (Motors are a prime example—they employ loops of wire and are considered in the next section.) Magnetohydrodynamics (MHD) is the technical name given to a clever application where magnetic force pumps fluids without moving mechanical parts. (See Figure 3.)

**Figure 3.** Magnetohydrodynamics. The magnetic force on the current passed through this fluid can be used as a nonmechanical pump.

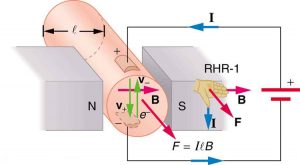
A strong magnetic field is applied across a tube and a current is passed through the fluid at right angles to the field, resulting in a force on the fluid parallel to the tube axis as shown. The absence of moving parts makes this attractive for moving a hot, chemically active substance, such as the liquid sodium employed in some nuclear reactors. Experimental artificial hearts are testing with this technique for pumping blood, perhaps circumventing the adverse effects of mechanical pumps. (Cell membranes, however, are affected by the large fields needed in MHD, delaying its practical application in humans.) MHD propulsion for nuclear submarines has been proposed, because it could be considerably quieter than conventional propeller drives. The deterrent value of nuclear submarines is based on their ability to hide and survive a first or second nuclear strike. As we slowly disassemble our nuclear weapons arsenals, the submarine branch will be the last to be decommissioned because of this ability (See Figure 4.) Existing MHD drives are heavy and inefficient—much development work is needed.

**Figure 4.** An MHD propulsion system in a nuclear submarine could produce significantly less turbulence than propellers and allow it to run more silently. The development of a silent drive submarine was dramatized in the book and the film *The Hunt for Red October*.

Section Summary

The magnetic force on current-carrying conductors is given by

$F = I l B \sin{\theta}$ ,

where $I$ is the current, $l$ is the length of a straight conductor in a uniform magnetic field $B$ , and $\theta$ is the angle between $I$ and $B$ . The force follows RHR-1 with the thumb in the direction of $I$ .

Homework

10. When is the force on a current carrying wire the strongest?

11. Determine the field from the force on a wire.

12. Determine the angle between a wire and the field.

License

Icon for the Creative Commons Attribution 4.0 International License

Physics 132: What is an Electron? What is Light? by Roger Hinrichs, Paul Peter Urone, Paul Flowers, Edward J. Neth, William R. Robinson, Klaus Theopold, Richard Langley, Julianne Zedalis, John Eggebrecht, and E.F. Redish is licensed under a Creative Commons Attribution 4.0 International License, except where otherwise noted.

Magnetic Fields and Magnetic Field Lines

Section Summary

Magnetic Field Strength: Force on a Moving Charge in a Magnetic Field

Right Hand Rule 1

Section Summary

Magnetic Force on a Current-Carrying Conductor

Section Summary

License

Share This Book