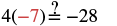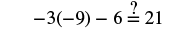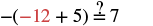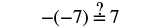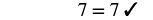Appendix G: Algebra Review
Izabela Mazur
Simplify Expressions by Combining Like Terms
Learning Objectives
By the end of this section, you will be able to:
- Evaluate algebraic expressions
- Identify terms, coefficients, and like terms
- Simplify expressions by combining like terms
- Translate word phrases to algebraic expressions
We can simplify an expression by combining the like terms. What do you think ![]() would simplify to? If you thought
would simplify to? If you thought ![]() , you would be right!
, you would be right!
We can see why this works by writing both terms as addition problems.
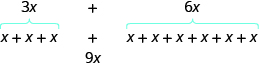
Add the coefficients and keep the same variable. It doesn’t matter what ![]() is. If you have
is. If you have ![]() of something and add
of something and add ![]() more of the same thing, the result is
more of the same thing, the result is ![]() of them. For example,
of them. For example, ![]() oranges plus
oranges plus ![]() oranges is
oranges is ![]() oranges. We will discuss the mathematical properties behind this later.
oranges. We will discuss the mathematical properties behind this later.
The expression ![]() has only two terms. When an expression contains more terms, it may be helpful to rearrange the terms so that like terms are together. The Commutative Property of Addition says that we can change the order of addends without changing the sum. So we could rearrange the following expression before combining like terms.
has only two terms. When an expression contains more terms, it may be helpful to rearrange the terms so that like terms are together. The Commutative Property of Addition says that we can change the order of addends without changing the sum. So we could rearrange the following expression before combining like terms.

Now it is easier to see the like terms to be combined.
HOW TO: Combine like terms
- Identify like terms.
- Rearrange the expression so like terms are together.
- Add the coefficients of the like terms.
EXAMPLE 9
Simplify the expression: ![]() .
.
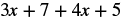 |
|
| Identify the like terms. | 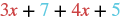 |
| Rearrange the expression, so the like terms are together. |  |
| Add the coefficients of the like terms. | 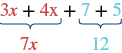 |
| The original expression is simplified to… |  |
TRY IT 9.1
Simplify:
![]()
Show Answer
16x + 17
TRY IT 9.2
Simplify:
![]()
Show Answer
17y + 7
EXAMPLE 10
Simplify the expression: ![]() .
.
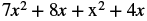 |
|
| Identify the like terms. | 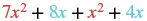 |
| Rearrange the expression so like terms are together. | 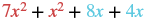 |
| Add the coefficients of the like terms. | 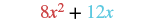 |
These are not like terms and cannot be combined. So ![]() is in simplest form.
is in simplest form.
TRY IT 10.1
Simplify:
![]()
Show Answer
4x2 + 14x
TRY IT 10.2
Simplify:
![]()
Show Answer
12y2 + 15y
Key Takeaways
For combining like terms:
- Identify like terms.
- Rearrange the expression so like terms are together.
- Add the coefficients of the like terms.
Solve Equations Using the Subtraction and Addition Properties of Equality
Learning Objectives
By the end of this section, you will be able to:
- Solve equations using the Subtraction and Addition Properties of Equality
- Solve equations that need to be simplified
- Translate an equation and solve
- Translate and solve applications
We are now ready to “get to the good stuff.” You have the basics down and are ready to begin one of the most important topics in algebra: solving equations. The applications are limitless and extend to all careers and fields. Also, the skills and techniques you learn here will help improve your critical thinking and problem-solving skills. This is a great benefit of studying mathematics and will be useful in your life in ways you may not see right now.
Solving an equation is like discovering the answer to a puzzle. The purpose in solving an equation is to find the value or values of the variable that make each side of the equation the same. Any value of the variable that makes the equation true is called a solution to the equation. It is the answer to the puzzle.
Solution of an Equation
A solution of an equation is a value of a variable that makes a true statement when substituted into the equation.
The steps to determine if a value is a solution to an equation are listed here.
HOW TO: Determine whether a number is a solution to an equation.
- Substitute the number for the variable in the equation.
- Simplify the expressions on both sides of the equation.
- Determine whether the resulting equation is true.
- If it is true, the number is a solution.
- If it is not true, the number is not a solution.
EXAMPLE 1
Determine whether ![]() is a solution for
is a solution for ![]() .
.
Solution
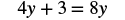 |
|
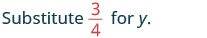 |
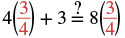 |
| Multiply. | 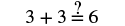 |
| Add. |  |
Since ![]() results in a true equation,
results in a true equation, ![]() is a solution to the equation
is a solution to the equation ![]() .
.
TRY IT 1.1
Is ![]() a solution for
a solution for ![]()
Show answer
no
TRY IT 1.2
Is ![]() a solution for
a solution for ![]()
Show answer
no
In that section,we will model how the Subtraction and Addition Properties work and then we will apply them to solve equations.
Subtraction Property of Equality
For all real numbers ![]() , and
, and ![]() , if
, if ![]() , then
, then ![]() .
.
Addition Property of Equality
For all real numbers ![]() , and
, and ![]() , if
, if ![]() , then
, then ![]() .
.
When you add or subtract the same quantity from both sides of an equation, you still have equality.
We will introduce the Subtraction Property of Equality by modeling equations with envelopes and counters. (Figure .1) models the equation ![]() .
.

The goal is to isolate the variable on one side of the equation. So we ‘took away’ ![]() from both sides of the equation and found the solution
from both sides of the equation and found the solution ![]() .
.
Some people picture a balance scale, as in (Figure .2), when they solve equations.
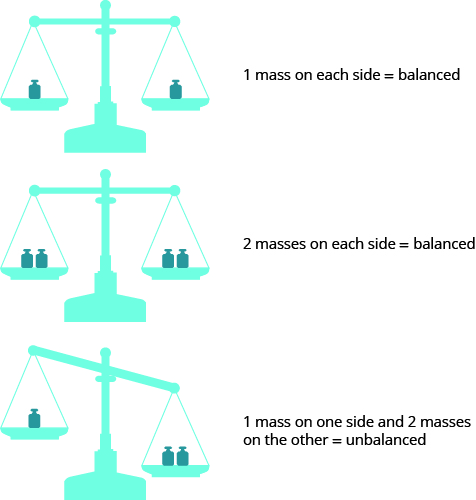
The quantities on both sides of the equal sign in an equation are equal, or balanced. Just as with the balance scale, whatever you do to one side of the equation you must also do to the other to keep it balanced.
Let’s see how to use Subtraction and Addition Properties of Equality to solve equations. We need to isolate the variable on one side of the equation. And we check our solutions by substituting the value into the equation to make sure we have a true statement.
EXAMPLE 2
Solve: ![]() .
.
Solution
To isolate ![]() , we undo the addition of
, we undo the addition of ![]() by using the Subtraction Property of Equality.
by using the Subtraction Property of Equality.
 |
||
| Subtract 11 from each side to “undo” the addition. | 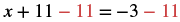 |
|
| Simplify. | 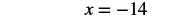 |
|
| Check: | 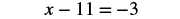 |
|
| Substitute |
 |
|
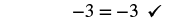 |
||
Since ![]() makes
makes ![]() a true statement, we know that it is a solution to the equation.
a true statement, we know that it is a solution to the equation.
TRY IT 2.1
Solve: ![]() .
.
Show answer
x = −16
TRY IT 2.2
Solve: ![]() .
.
Show answer
x = −20
In the original equation in the previous example, ![]() was added to the
was added to the ![]() , so we subtracted
, so we subtracted ![]() to ‘undo’ the addition. In the next example, we will need to ‘undo’ subtraction by using the Addition Property of Equality.
to ‘undo’ the addition. In the next example, we will need to ‘undo’ subtraction by using the Addition Property of Equality.
EXAMPLE 3
Solve: ![]() .
.
Solution
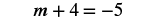 |
||
| Add 4 to each side to “undo” the subtraction. | 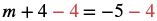 |
|
| Simplify. | 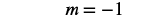 |
|
| Check: | 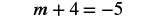 |
|
| Substitute |
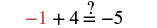 |
|
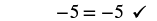 |
||
| The solution to |
||
TRY IT 3.1
Solve: ![]() .
.
Show answer
−1
TRY IT 3.2
Solve: ![]() .
.
Show answer
−4
Now let’s solve equations with fractions.
EXAMPLE 4
Solve: ![]() .
.
Solution
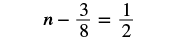 |
||
| Use the Addition Property of Equality. | 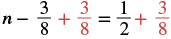 |
|
| Find the LCD to add the fractions on the right. |  |
|
| Simplify | 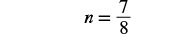 |
|
| Check: | 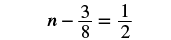 |
|
 |
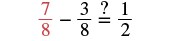 |
|
| Subtract. |  |
|
| Simplify. | 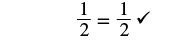 |
|
| The solution checks. | ||
TRY IT 4.1
Solve: ![]() .
.
Show answer
![]()
TRY IT 4.2
Solve: ![]() .
.
Show answer
![]()
Let’s solve equations that contained decimals.
EXAMPLE 5
Solve ![]() .
.
Solution
 |
||
| Use the Addition Property of Equality. | 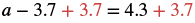 |
|
| Add. | 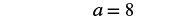 |
|
| Check: | 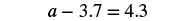 |
|
| Substitute |
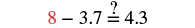 |
|
| Simplify. | 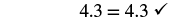 |
|
| The solution checks. | ||
TRY IT 5.1
Solve: ![]() .
.
Show answer
b = 6.4
TRY IT 5.2
Solve: ![]() .
.
Show answer
c = 14
Solve Equations That Need to Be Simplified
In the examples up to this point, we have been able to isolate the variable with just one operation. Many of the equations we encounter in algebra will take more steps to solve. Usually, we will need to simplify one or both sides of an equation before using the Subtraction or Addition Properties of Equality. You should always simplify as much as possible before trying to isolate the variable.
EXAMPLE 6
Solve: ![]() .
.
Solution
The left side of the equation has an expression that we should simplify before trying to isolate the variable.
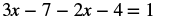 |
|
| Rearrange the terms, using the Commutative Property of Addition. | 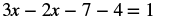 |
| Combine like terms. | 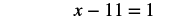 |
| Add 11 to both sides to isolate |
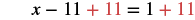 |
| Simplify. |  |
| Check. Substitute 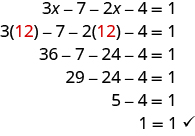 |
The solution checks.
TRY IT 6.1
Solve: ![]() .
.
Show answer
y = 15
TRY IT 6.2
Solve: ![]() .
.
Show answer
z = 2
EXAMPLE 7
Solve: ![]() .
.
Solution
The left side of the equation has an expression that we should simplify.
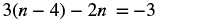 |
|
| Distribute on the left. | 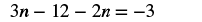 |
| Use the Commutative Property to rearrange terms. | 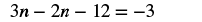 |
| Combine like terms. | 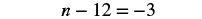 |
| Isolate n using the Addition Property of Equality. | 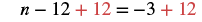 |
| Simplify. | 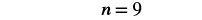 |
| Check. Substitute  The solution checks. |
TRY IT 7.1
Solve: ![]() .
.
Show answer
p = 5
TRY IT 7.2
Solve: ![]() .
.
Show answer
q = −16
EXAMPLE 8
Solve: ![]() .
.
Solution
Both sides of the equation have expressions that we should simplify before we isolate the variable.
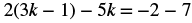 |
|
| Distribute on the left, subtract on the right. | 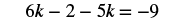 |
| Use the Commutative Property of Addition. | 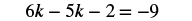 |
| Combine like terms. | 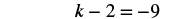 |
| Undo subtraction by using the Addition Property of Equality. | 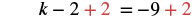 |
| Simplify. |  |
| Check. Let |
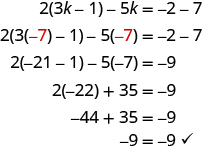 |
| The solution checks. |
TRY IT 8.1
Solve: ![]() .
.
Show answer
h = −1
TRY IT 8.2
Solve: ![]() .
.
Show answer
x = 1
Solve Equations Using the Division and Multiplication Properties of Equality
Learning Objectives
By the end of this section, you will be able to:
- Solve equations using the Division and Multiplication Properties of Equality
- Solve equations that need to be simplified
You may have noticed that all of the equations we have solved so far have been of the form ![]() or
or ![]() . We were able to isolate the variable by adding or subtracting the constant term on the side of the equation with the variable. Now we will see how to solve equations that have a variable multiplied by a constant and so will require division to isolate the variable.
. We were able to isolate the variable by adding or subtracting the constant term on the side of the equation with the variable. Now we will see how to solve equations that have a variable multiplied by a constant and so will require division to isolate the variable.
Let’s look at our puzzle again with the envelopes and counters in (Figure 1).
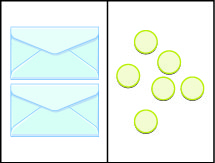
In the illustration there are two identical envelopes that contain the same number of counters. Remember, the left side of the workspace must equal the right side, but the counters on the left side are “hidden” in the envelopes. So how many counters are in each envelope?
How do we determine the number? We have to separate the counters on the right side into two groups of the same size to correspond with the two envelopes on the left side. The 6 counters divided into 2 equal groups gives 3 counters in each group (since ![]() ).
).
What equation models the situation shown in (Figure 2)? There are two envelopes, and each contains ![]() counters. Together, the two envelopes must contain a total of 6 counters.
counters. Together, the two envelopes must contain a total of 6 counters.
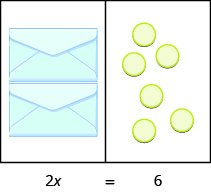
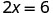 |
|
| If we divide both sides of the equation by 2, as we did with the envelopes and counters, | 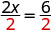 |
| we get: | 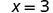 |
We found that each envelope contains 3 counters. Does this check? We know ![]() , so it works! Three counters in each of two envelopes does equal six!
, so it works! Three counters in each of two envelopes does equal six!
This example leads to the Division Property of Equality.
Division and Multiplication Properties of Equality
Division Property of Equality: For all real numbers ![]() , and
, and ![]() , if
, if ![]() , then
, then ![]() .
.
Multiplication Property of Equality: For all real numbers ![]() , if
, if ![]() , then
, then ![]() .
.
When you divide or multiply both sides of an equation by the same quantity, you still have equality.
Let’s review how these properties of equality can be applied in order to solve equations. Remember, the goal is to ‘undo’ the operation on the variable. In the example below the variable is multiplied by ![]() , so we will divide both sides by
, so we will divide both sides by ![]() to ‘undo’ the multiplication.
to ‘undo’ the multiplication.
EXAMPLE 1
Solve: ![]() .
.
Solution
We use the Division Property of Equality to divide both sides by ![]() .
.
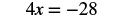 |
|
| Divide both sides by 4 to undo the multiplication. | 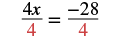 |
| Simplify. |  |
| Check your answer. Let |
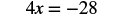
|
Since this is a true statement, ![]() is a solution to
is a solution to ![]() .
.
TRY IT 1.1
Solve: ![]() .
.
Show answer
y = −16
TRY IT 1.2
Solve: ![]() .
.
Show answer
z = −13
In the previous example, to ‘undo’ multiplication, we divided. How do you think we ‘undo’ division?
EXAMPLE 2
Solve: ![]() .
.
Solution
Here ![]() is divided by
is divided by ![]() . We can multiply both sides by
. We can multiply both sides by ![]() to isolate
to isolate ![]() .
.
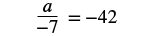 |
|
| Multiply both sides by |
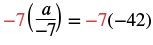  |
| Simplify. |  |
| Check your answer. Let |
|
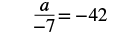 |
|
 |
|
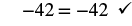 |
TRY IT 2.1
Solve: ![]() .
.
Show answer
b = 144
TRY IT 2.2
Solve: ![]() .
.
Show answer
c = 128
EXAMPLE 3
Solve: ![]() .
.
Solution
Remember ![]() is equivalent to
is equivalent to ![]() .
.
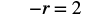 |
||
| Rewrite |
 |
|
| Divide both sides by |
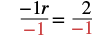 |
|
 |
||
| Check. | 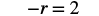 |
|
| Substitute |
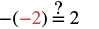 |
|
| Simplify. | 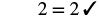 |
|
We see that there are two other ways to solve ![]() .
.
We could multiply both sides by ![]() .
.
We could take the opposite of both sides.
TRY IT 3.1
Solve: ![]() .
.
Show answer
k = −8
TRY IT 3.2
Solve: ![]() .
.
Show answer
g = −3
EXAMPLE 4
Solve: ![]() .
.
Solution
Since the product of a number and its reciprocal is ![]() , our strategy will be to isolate
, our strategy will be to isolate ![]() by multiplying by the reciprocal of
by multiplying by the reciprocal of ![]() .
.
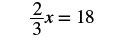 |
|
| Multiply by the reciprocal of |
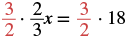 |
| Reciprocals multiply to one. |  |
| Multiply. | 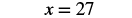 |
| Check your answer. Let |
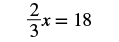
|
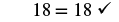 |
Notice that we could have divided both sides of the equation ![]() by
by ![]() to isolate
to isolate ![]() . While this would work, multiplying by the reciprocal requires fewer steps.
. While this would work, multiplying by the reciprocal requires fewer steps.
TRY IT 4.1
Solve: ![]() .
.
Show answer
n = 35
TRY IT 4.2
Solve: ![]() .
.
Show answer
y = 18
Solve Equations That Need to be Simplified
Many equations start out more complicated than the ones we’ve just solved. First, we need to simplify both sides of the equation as much as possible
EXAMPLE 5
Solve: ![]() .
.
Solution
Start by combining like terms to simplify each side.
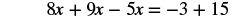 |
|
| Combine like terms. | 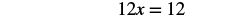 |
| Divide both sides by 12 to isolate x. | 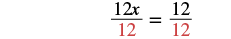 |
| Simplify. |  |
| Check your answer. Let |
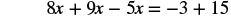
|
TRY IT 5.1
Solve: ![]() .
.
Show answer
x = 2
TRY IT 5.2
Solve: ![]() .
.
Show answer
n = −5
EXAMPLE 6
Solve: ![]() .
.
Solution
Simplify each side by combining like terms.
 |
|
| Simplify each side. |  |
| Divide both sides by 3 to isolate y. |  |
| Simplify. |  |
| Check your answer. Let |
|
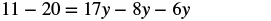 |
|
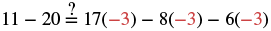 |
|
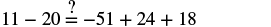 |
|
 |
Notice that the variable ended up on the right side of the equal sign when we solved the equation. You may prefer to take one more step to write the solution with the variable on the left side of the equal sign.
TRY IT 6.1
Solve: ![]() .
.
Show answer
c = −3
TRY IT 6.2
Solve: ![]() .
.
Show answer
![]()
EXAMPLE 7
Solve: ![]() .
.
Solution
Remember—always simplify each side first.
 |
|
| Distribute. | 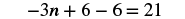 |
| Simplify. | 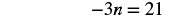 |
| Divide both sides by -3 to isolate n. | 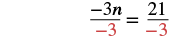  |
| Check your answer. Let |
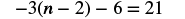
|
TRY IT 7.1
Solve: ![]() .
.
Show answer
n = −6
TRY IT 7.2
Solve: ![]() .
.
Show answer
n = −5
Key Takeaways
- Division and Multiplication Properties of Equality
- Division Property of Equality: For all real numbers a, b, c, and
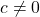 , if
, if  , then
, then 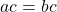 .
. - Multiplication Property of Equality: For all real numbers a, b, c, if
 , then
, then 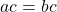 .
.
- Division Property of Equality: For all real numbers a, b, c, and
expage]
Learning Objectives
By the end of this section, you will be able to:
- Solve an equation with constants on both sides
- Solve an equation with variables on both sides
- Solve an equation with variables and constants on both sides
- Solve equations using a general strategy
Solve an Equation with Constants on Both Sides
You may have noticed that in all the equations we have solved so far, all the variable terms were on only one side of the equation with the constants on the other side. This does not happen all the time—so now we’ll see how to solve equations where the variable terms and/or constant terms are on both sides of the equation.
Our strategy will involve choosing one side of the equation to be the variable side, and the other side of the equation to be the constant side. Then, we will use the Subtraction and Addition Properties of Equality, step by step, to get all the variable terms together on one side of the equation and the constant terms together on the other side.
By doing this, we will transform the equation that started with variables and constants on both sides into the form ![]() . We already know how to solve equations of this form by using the Division or Multiplication Properties of Equality.
. We already know how to solve equations of this form by using the Division or Multiplication Properties of Equality.
EXAMPLE 1
Solve: ![]() .
.
Solution
In this equation, the variable is only on the left side. It makes sense to call the left side the variable side. Therefore, the right side will be the constant side. We’ll write the labels above the equation to help us remember what goes where.
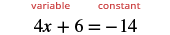 |
||
| Since the left side is the variable side, the 6 is out of place. We must “undo” adding 6 by subtracting 6, and to keep the equality we must subtract 6 from both sides. Use the Subtraction Property of Equality. | 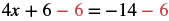 |
|
| Simplify. | 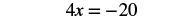 |
|
| Now all the |
||
| Use the Division Property of Equality. |  |
|
| Simplify. | 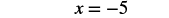 |
|
| Check: | 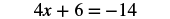 |
|
| Let |
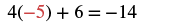 |
|
 |
||
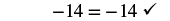 |
||
TRY IT 1.1
Solve: ![]() .
.
Show answer
x = −4
TRY IT 1.2
Solve: ![]() .
.
Show answer
a = −8
EXAMPLE 1.2
Solve: ![]() .
.
Solution
Notice that the variable is only on the left side of the equation, so this will be the variable side and the right side will be the constant side. Since the left side is the variable side, the ![]() is out of place. It is subtracted from the
is out of place. It is subtracted from the ![]() , so to ‘undo’ subtraction, add
, so to ‘undo’ subtraction, add ![]() to both sides.
to both sides.
 |
||
| Add 7 to both sides. | 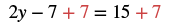 |
|
| Simplify. | 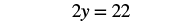 |
|
| The variables are now on one side and the constants on the other. | ||
| Divide both sides by 2. |  |
|
| Simplify. | 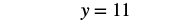 |
|
| Check: | 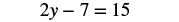 |
|
| Substitute: |
 |
|
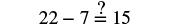 |
||
 |
||
TRY IT 2.1
Solve: ![]() .
.
Show answer
y = 5
TRY IT 2.2
Solve: ![]() .
.
Show answer
m = 9
Solve an Equation with Variables on Both Sides
What if there are variables on both sides of the equation? We will start like we did above—choosing a variable side and a constant side, and then use the Subtraction and Addition Properties of Equality to collect all variables on one side and all constants on the other side. Remember, what you do to the left side of the equation, you must do to the right side too.
EXAMPLE 3
Solve: ![]() .
.
Here the variable, ![]() , is on both sides, but the constants appear only on the right side, so let’s make the right side the “constant” side. Then the left side will be the “variable” side.
, is on both sides, but the constants appear only on the right side, so let’s make the right side the “constant” side. Then the left side will be the “variable” side.
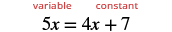 |
||
| We don’t want any variables on the right, so subtract the |
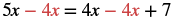 |
|
| Simplify. | 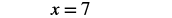 |
|
| We have all the variables on one side and the constants on the other. We have solved the equation. | ||
| Check: | 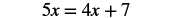 |
|
| Substitute 7 for |
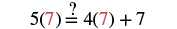 |
|
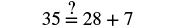 |
||
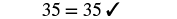 |
||
TRY IT 3.1
Solve: ![]() .
.
Show answer
n = 10
TRY IT 3.2
Solve: ![]() .
.
Show answer
c = 1
EXAMPLE 4
Solve: ![]() .
.
Solution
The only constant, ![]() , is on the left side of the equation and variable,
, is on the left side of the equation and variable, ![]() , is on both sides. Let’s leave the constant on the left and collect the variables to the right.
, is on both sides. Let’s leave the constant on the left and collect the variables to the right.
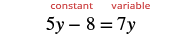 |
|
| Subtract |
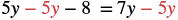 |
| Simplify. | 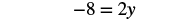 |
| We have the variables on the right and the constants on the left. Divide both sides by 2. | 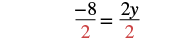 |
| Simplify. | 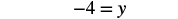 |
| Rewrite with the variable on the left. | 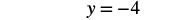 |
| Check: Let |
|
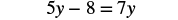 |
|
 |
|
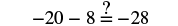 |
|
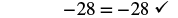 |
TRY IT 4.1
Solve: ![]() .
.
Show answer
p = −7
TRY IT 4.2
Solve: ![]() .
.
Show answer
m = −3
EXAMPLE 5
Solve: ![]() .
.
Solution
The only constant, ![]() , is on the right, so let the left side be the variable side.
, is on the right, so let the left side be the variable side.
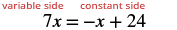 |
|
| Remove the |
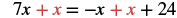 |
| Simplify. | 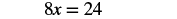 |
| All the variables are on the left and the constants are on the right. Divide both sides by 8. | 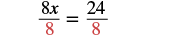 |
| Simplify. | 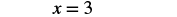 |
| Check: Substitute |
|
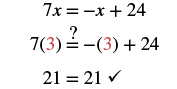 |
TRY IT 5.1
Solve: ![]() .
.
Show answer
j = 2
TRY IT 5.2
Solve: ![]() .
.
Show answer
h = 1
Solve Equations with Variables and Constants on Both Sides
The next example will be the first to have variables and constants on both sides of the equation. As we did before, we’ll collect the variable terms to one side and the constants to the other side.
EXAMPLE 6
Solve: ![]() .
.
Solution
Start by choosing which side will be the variable side and which side will be the constant side. The variable terms are ![]() and
and ![]() . Since
. Since ![]() is greater than
is greater than ![]() , make the left side the variable side and so the right side will be the constant side.
, make the left side the variable side and so the right side will be the constant side.
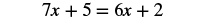 |
|
| Collect the variable terms to the left side by subtracting |
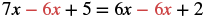 |
| Simplify. | 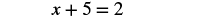 |
| Now, collect the constants to the right side by subtracting 5 from both sides. | 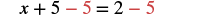 |
| Simplify. | 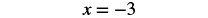 |
| The solution is |
|
| Check: Let |
|
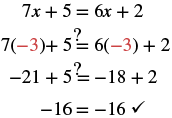 |
TRY IT 6.1
Solve: ![]() .
.
Show answer
x = −1
TRY IT 6.2
Solve: ![]() .
.
Show answer
y = 4
We’ll summarize the steps we took so you can easily refer to them.
HOW TO: Solve an Equation with Variables and Constants on Both Sides
- Choose one side to be the variable side and then the other will be the constant side.
- Collect the variable terms to the variable side, using the Addition or Subtraction Property of Equality.
- Collect the constants to the other side, using the Addition or Subtraction Property of Equality.
- Make the coefficient of the variable
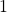 , using the Multiplication or Division Property of Equality.
, using the Multiplication or Division Property of Equality. - Check the solution by substituting it into the original equation.
It is a good idea to make the variable side the one in which the variable has the larger coefficient. This usually makes the arithmetic easier.
EXAMPLE 7
Solve: ![]() .
.
Solution
We have ![]() on the left and
on the left and ![]() on the right. Since
on the right. Since ![]() >
> ![]() , make the left side the “variable” side.
, make the left side the “variable” side.
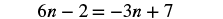 |
|
| We don’t want variables on the right side—add |
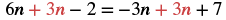 |
| Combine like terms. | 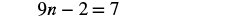 |
| We don’t want any constants on the left side, so add 2 to both sides. | 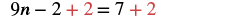 |
| Simplify. | 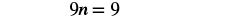 |
| The variable term is on the left and the constant term is on the right. To get the coefficient of |
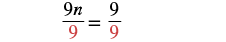 |
| Simplify. | 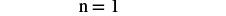 |
| Check: Substitute 1 for |
 |
TRY IT 7.1
Solve: ![]() .
.
Show answer
q = 1
TRY IT 7.2
Solve: ![]() .
.
Show answer
n = 1
EXAMPLE 8
Solve: ![]() .
.
Solution
This equation has ![]() on the left and
on the left and ![]() on the right. Since
on the right. Since ![]() >
> ![]() , make the right side the variable side and the left side the constant side.
, make the right side the variable side and the left side the constant side.
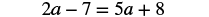 |
|
| Subtract |
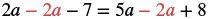 |
| Combine like terms. | 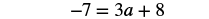 |
| Subtract 8 from both sides to remove the constant from the right. | 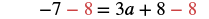 |
| Simplify. | 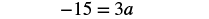 |
| Divide both sides by 3 to make 1 the coefficient of |
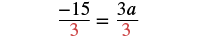 |
| Simplify. | 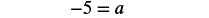 |
| Check: Let |
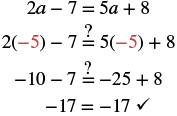 |
Note that we could have made the left side the variable side instead of the right side, but it would have led to a negative coefficient on the variable term. While we could work with the negative, there is less chance of error when working with positives. The strategy outlined above helps avoid the negatives!
TRY IT 8.1
Solve: ![]() .
.
Show answer
a = −5
TRY IT 8.2
Solve: ![]() .
.
Show answer
k = −6
To solve an equation with fractions, we still follow the same steps to get the solution.
EXAMPLE 9
Solve: ![]() .
.
Solution
Since ![]() >
> ![]() , make the left side the variable side and the right side the constant side.
, make the left side the variable side and the right side the constant side.
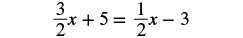 |
|
| Subtract |
 |
| Combine like terms. |  |
| Subtract 5 from both sides. |  |
| Simplify. |  |
| Check: Let |
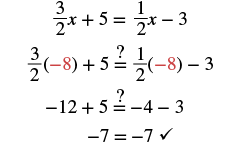 |
TRY IT 9.1
Solve: ![]() .
.
Show answer
x = 10
TRY IT 9.2
Solve: ![]() .
.
Show answer
y = −3
We follow the same steps when the equation has decimals, too.
EXAMPLE 10
Solve: ![]() .
.
Solution
Since ![]() >
> ![]() , make the left side the variable side and the right side the constant side.
, make the left side the variable side and the right side the constant side.
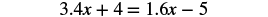 |
|
| Subtract |
 |
| Combine like terms. | 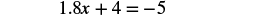 |
| Subtract 4 from both sides. | 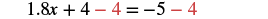 |
| Simplify. | 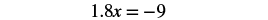 |
| Use the Division Property of Equality. | 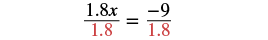 |
| Simplify. | 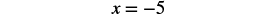 |
| Check: Let |
 |
TRY IT 10.1
Solve: ![]() .
.
Show answer
x = −5
TRY IT 10.2
Solve: ![]() .
.
Show answer
y = −5
Solve Equations Using a General Strategy
Each of the first few sections of this chapter has dealt with solving one specific form of a linear equation. It’s time now to lay out an overall strategy that can be used to solve any linear equation. We call this the general strategy. Some equations won’t require all the steps to solve, but many will. Simplifying each side of the equation as much as possible first makes the rest of the steps easier.
HOW TO: Use a General Strategy for Solving Linear Equations
- Simplify each side of the equation as much as possible. Use the Distributive Property to remove any parentheses. Combine like terms.
- Collect all the variable terms to one side of the equation. Use the Addition or Subtraction Property of Equality.
- Collect all the constant terms to the other side of the equation. Use the Addition or Subtraction Property of Equality.
- Make the coefficient of the variable term to equal to
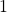 . Use the Multiplication or Division Property of Equality. State the solution to the equation.
. Use the Multiplication or Division Property of Equality. State the solution to the equation. - Check the solution. Substitute the solution into the original equation to make sure the result is a true statement.
EXAMPLE 11
Solve: ![]() .
.
Solution
 |
|
| Simplify each side of the equation as much as possible. Use the Distributive Property. |
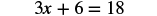 |
| Collect all variable terms on one side of the equation—all |
|
| Collect constant terms on the other side of the equation. Subtract 6 from each side |
 |
| Simplify. | 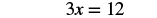 |
| Make the coefficient of the variable term equal to 1. Divide each side by 3. | 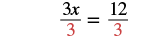 |
| Simplify. | 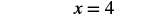 |
| Check: Let |
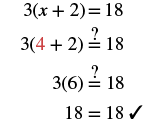 |
TRY IT 11.1
Solve: ![]() .
.
Show answer
x = 4
TRY IT 11.2
Solve: ![]() .
.
Show answer
y = 1
EXAMPLE 12
Solve: ![]() .
.
Solution
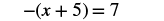 |
|
| Simplify each side of the equation as much as possible by distributing. The only |
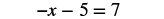 |
| Add 5 to both sides to get all constant terms on the right side of the equation. | 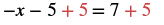 |
| Simplify. | 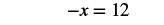 |
| Make the coefficient of the variable term equal to 1 by multiplying both sides by -1. | 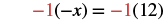 |
| Simplify. | 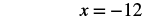 |
| Check: Let |
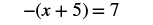
|
TRY IT 12.1
Solve: ![]() .
.
Show answer
y = −6
TRY IT 12.2
Solve: ![]() .
.
Show answer
z = 8
EXAMPLE 13
Solve: ![]() .
.
Solution
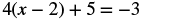 |
|
| Simplify each side of the equation as much as possible. Distribute. |
 |
| Combine like terms | 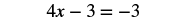 |
| The only |
|
| Add 3 to both sides to get all constant terms on the other side of the equation. | 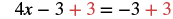 |
| Simplify. | 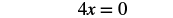 |
| Make the coefficient of the variable term equal to 1 by dividing both sides by 4. |  |
| Simplify. | 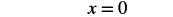 |
| Check: Let |
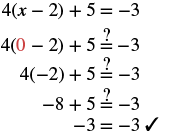 |
TRY IT 13.1
Solve: ![]() .
.
Show answer
a = 2
TRY IT 13.2
Solve: ![]() .
.
Show answer
n = 2
EXAMPLE 14
Solve: ![]() .
.
Solution
Be careful when distributing the negative.
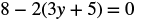 |
|
| Simplify—use the Distributive Property. |  |
| Combine like terms. | 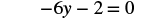 |
| Add 2 to both sides to collect constants on the right. |  |
| Simplify. | 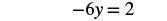 |
| Divide both sides by −6. |  |
| Simplify. | 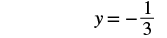 |
| Check: Let |
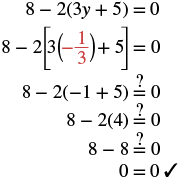 |
TRY IT 14.1
Solve: ![]() .
.
Show answer
![]()
TRY IT 14.2
Solve: ![]() .
.
Show answer
![]()
EXAMPLE 15
Solve: ![]() .
.
Solution
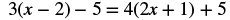 |
|
| Distribute. | 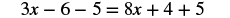 |
| Combine like terms. | 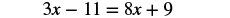 |
| Subtract |
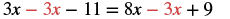 |
| Simplify. | 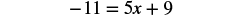 |
| Subtract 9 to get the constants on the left. | 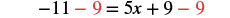 |
| Simplify. |  |
| Divide by 5. | 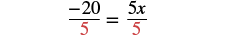 |
| Simplify. | 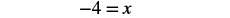 |
| Check: Substitute: |
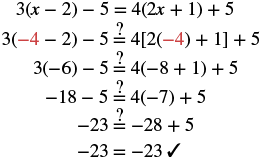 |
TRY IT 14.1
Solve: ![]() .
.
Show answer
p = −2
TRY IT 14.2
Solve: ![]() .
.
Show answer
q = −8
EXAMPLE 15
Solve: ![]() .
.
Solution
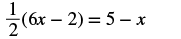 |
|
| Distribute. | 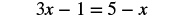 |
| Add |
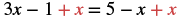 |
| Simplify. | 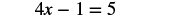 |
| Add 1 to get constants on the right. | 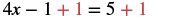 |
| Simplify. |  |
| Divide by 4. | 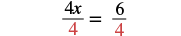 |
| Simplify. | 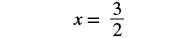 |
| Check: Let |
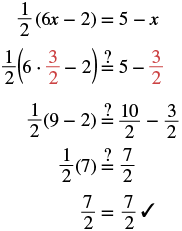 |
TRY IT 15.1
Solve: ![]() .
.
Show answer
u = 2
TRY IT 15.2
Solve: ![]() .
.
Show answer
x = 4
In many applications, we will have to solve equations with decimals. The same general strategy will work for these equations.
EXAMPLE 16
Solve: ![]() .
.
Solution
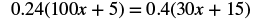 |
|
| Distribute. | 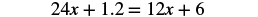 |
| Subtract |
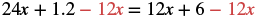 |
| Simplify. |  |
| Subtract 1.2 to get the constants to the right. | 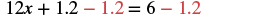 |
| Simplify. |  |
| Divide. | 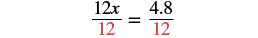 |
| Simplify. | 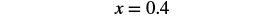 |
| Check: Let |
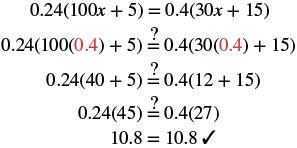 |
TRY IT 16.1
Solve: ![]() .
.
Show answer
1
TRY IT 16.2
Solve: ![]() .
.
Show answer
−1
Key Takeaways
- Solve an equation with variables and constants on both sides
- Choose one side to be the variable side and then the other will be the constant side.
- Collect the variable terms to the variable side, using the Addition or Subtraction Property of Equality.
- Collect the constants to the other side, using the Addition or Subtraction Property of Equality.
- Make the coefficient of the variable 1, using the Multiplication or Division Property of Equality.
- Check the solution by substituting into the original equation.
- General strategy for solving linear equations
- Simplify each side of the equation as much as possible. Use the Distributive Property to remove any parentheses. Combine like terms.
- Collect all the variable terms to one side of the equation. Use the Addition or Subtraction Property of Equality.
- Collect all the constant terms to the other side of the equation. Use the Addition or Subtraction Property of Equality.
- Make the coefficient of the variable term to equal to 1. Use the Multiplication or Division Property of Equality. State the solution to the equation.
- Check the solution. Substitute the solution into the original equation to make sure the result is a true statement.
Homework
- Algebra review (questions 5 and 6).
Attributions
This chapter has been adapted from “Evaluate, Simplify, and Translate Expressions” in Prealgebra (OpenStax) by Lynn Marecek, MaryAnne Anthony-Smith, and Andrea Honeycutt Mathis, which is under a CC BY 4.0 Licence. Adapted by Izabela Mazur. See the Copyright page for more information.