Appendix L: Triangles and the Pythagorean Theorem
Izabela Mazur
Learning Objectives
By the end of this section, you will be able to:
- Use the properties of angles
- Use the properties of triangles
- Use the Pythagorean Theorem
Use the Properties of Triangles
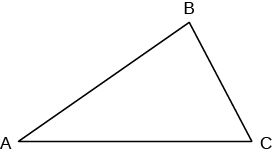
What do you already know about triangles? Triangle have three sides and three angles. Triangles are named by their vertices. The triangle in (Figure 5) is called ![]() , read ‘triangle
, read ‘triangle ![]() ’. We label each side with a lower case letter to match the upper case letter of the opposite vertex.
’. We label each side with a lower case letter to match the upper case letter of the opposite vertex.
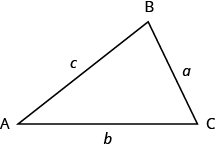
The three angles of a triangle are related in a special way. The sum of their measures is ![]() °.
°.
Sum of the Measures of the Angles of a Triangle
For any ![]() , the sum of the measures of the angles is
, the sum of the measures of the angles is ![]() °.
°.
EXAMPLE 3
The measures of two angles of a triangle are ![]() ° and
° and ![]() °. Find the measure of the third angle.
°. Find the measure of the third angle.
Solution
| Step 1. Read the problem. Draw the figure and label it with the given information. | 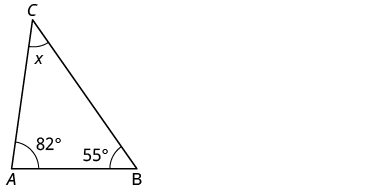 |
| Step 2. Identify what you are looking for. |  |
| Step 3. Name. Choose a variable to represent it. |  |
| Step 4. Translate. Write the appropriate formula and substitute. |
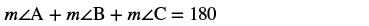 |
| Step 5. Solve the equation. | 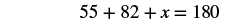 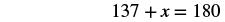  |
Step 6. Check: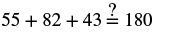 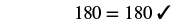 |
|
| Step 7. Answer the question. |  |
TRY IT 3.1
The measures of two angles of a triangle are ![]() ° and
° and ![]() °. Find the measure of the third angle.
°. Find the measure of the third angle.
Show answer
21°
TRY IT 3.2
A triangle has angles of ![]() ° and
° and ![]() °. Find the measure of the third angle.
°. Find the measure of the third angle.
Show answer
56°
Right Triangles
Some triangles have special names. We will look first at the right triangle. A right triangle has one ![]() ° angle, which is often marked with the symbol shown in (Figure 6).
° angle, which is often marked with the symbol shown in (Figure 6).
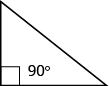
If we know that a triangle is a right triangle, we know that one angle measures ![]() ° so we only need the measure of one of the other angles in order to determine the measure of the third angle.
° so we only need the measure of one of the other angles in order to determine the measure of the third angle.
EXAMPLE 4
One angle of a right triangle measures ![]() °. What is the measure of the third angle?
°. What is the measure of the third angle?
Solution
| Step 1. Read the problem. Draw the figure and label it with the given information. | 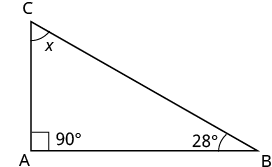 |
| Step 2. Identify what you are looking for. |  |
| Step 3. Name. Choose a variable to represent it. |  |
| Step 4. Translate. Write the appropriate formula and substitute. |
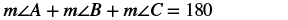 |
| Step 5. Solve the equation. | 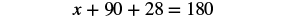 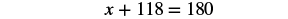  |
Step 6. Check: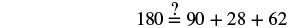 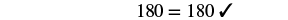 |
|
| Step 7. Answer the question. |  |
TRY IT 4.1
One angle of a right triangle measures ![]() °. What is the measure of the other angle?
°. What is the measure of the other angle?
Show answer
34°
TRY IT 4.2
One angle of a right triangle measures ![]() °. What is the measure of the other angle?
°. What is the measure of the other angle?
Show answer
45°
In the examples so far, we could draw a figure and label it directly after reading the problem. In the next example, we will have to define one angle in terms of another. So we will wait to draw the figure until we write expressions for all the angles we are looking for.
EXAMPLE 5
The measure of one angle of a right triangle is ![]() ° more than the measure of the smallest angle. Find the measures of all three angles.
° more than the measure of the smallest angle. Find the measures of all three angles.
Solution
| Step 1. Read the problem. | |
| Step 2. Identify what you are looking for. | the measures of all three angles |
| Step 3. Name. Choose a variable to represent it.
Now draw the figure and label it with the given information. |
   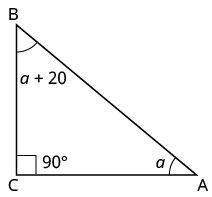 |
| Step 4. Translate. Write the appropriate formula and substitute into the formula. |
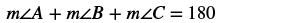 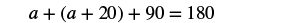 |
| Step 5. Solve the equation. | 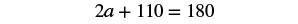 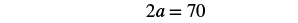 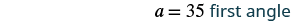     |
Step 6. Check: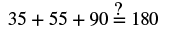 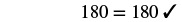 |
|
| Step 7. Answer the question. |  |
TRY IT 5.1
The measure of one angle of a right triangle is ![]() ° more than the measure of the smallest angle. Find the measures of all three angles.
° more than the measure of the smallest angle. Find the measures of all three angles.
Show answer
20°, 70°, 90°
TRY IT 5.2
The measure of one angle of a right triangle is ![]() ° more than the measure of the smallest angle. Find the measures of all three angles.
° more than the measure of the smallest angle. Find the measures of all three angles.
Show answer
30°, 60°, 90°
Use the Pythagorean Theorem
The Pythagorean Theorem is a special property of right triangles that has been used since ancient times. It is named after the Greek philosopher and mathematician Pythagoras who lived around ![]() BCE.
BCE.
Remember that a right triangle has a ![]() ° angle, which we usually mark with a small square in the corner. The side of the triangle opposite the
° angle, which we usually mark with a small square in the corner. The side of the triangle opposite the ![]() ° angle is called the hypotenuse, and the other two sides are called the legs. See (Figure 8).
° angle is called the hypotenuse, and the other two sides are called the legs. See (Figure 8).
In a right triangle, the side opposite the ![]() ° angle is called the hypotenuse and each of the other sides is called a leg.
° angle is called the hypotenuse and each of the other sides is called a leg.
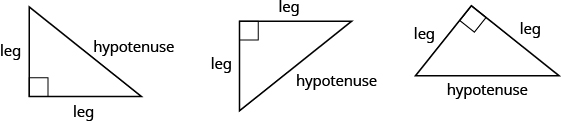
The Pythagorean Theorem tells how the lengths of the three sides of a right triangle relate to each other. It states that in any right triangle, the sum of the squares of the two legs equals the square of the hypotenuse.
The Pythagorean Theorem
In any right triangle ![]() ,
,
where ![]() is the length of the hypotenuse
is the length of the hypotenuse ![]() and
and ![]() are the lengths of the legs.
are the lengths of the legs.
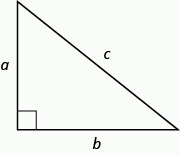
To solve problems that use the Pythagorean Theorem, we will need to find square roots. We defined the notation ![]() in this way:
in this way:
![]()
For example, we found that ![]() is
is ![]() because
because ![]() .
.
We will use this definition of square roots to solve for the length of a side in a right triangle.
EXAMPLE 7
Use the Pythagorean Theorem to find the length of the hypotenuse.
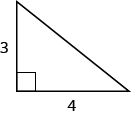
Solution
| Step 1. Read the problem. | |
| Step 2. Identify what you are looking for. | the length of the hypotenuse of the triangle |
| Step 3. Name. Choose a variable to represent it. | Let 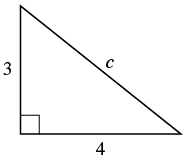 |
| Step 4. Translate. Write the appropriate formula. Substitute. |
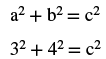 |
| Step 5. Solve the equation. | 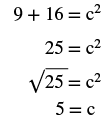 |
Step 6. Check: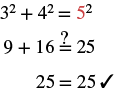 |
|
| Step 7. Answer the question. | The length of the hypotenuse is 5. |
TRY IT 7.1
Use the Pythagorean Theorem to find the length of the hypotenuse.
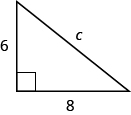
Show answer
10
TRY IT 7.2
Use the Pythagorean Theorem to find the length of the hypotenuse.
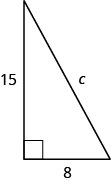
Show answer
17
EXAMPLE 8
Use the Pythagorean Theorem to find the length of the longer leg.

Solution
| Step 1. Read the problem. | |
| Step 2. Identify what you are looking for. | The length of the leg of the triangle |
| Step 3. Name. Choose a variable to represent it. | Let Label side b 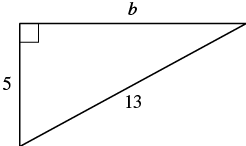 |
| Step 4. Translate. Write the appropriate formula. Substitute. |
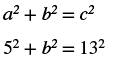 |
| Step 5. Solve the equation. Isolate the variable term. Use the definition of the square root. Simplify. |
 |
| Step 6. Check: | 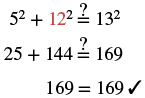 |
| Step 7. Answer the question. | The length of the leg is 12. |
TRY IT 8.1
Use the Pythagorean Theorem to find the length of the leg.
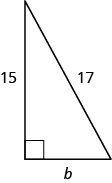
Show answer
8
TRY IT 8.2
Use the Pythagorean Theorem to find the length of the leg.
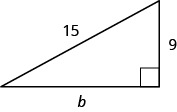
Show answer
12
EXAMPLE 9
Kelvin is building a gazebo and wants to brace each corner by placing a ![]() wooden bracket diagonally as shown. How far below the corner should he fasten the bracket if he wants the distances from the corner to each end of the bracket to be equal? Approximate to the nearest tenth of an inch.
wooden bracket diagonally as shown. How far below the corner should he fasten the bracket if he wants the distances from the corner to each end of the bracket to be equal? Approximate to the nearest tenth of an inch.
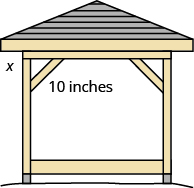
Solution
| Step 1. Read the problem. | |
| Step 2. Identify what you are looking for. | the distance from the corner that the bracket should be attached |
| Step 3. Name. Choose a variable to represent it. | Let x = the distance from the corner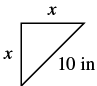 |
| Step 4. Translate. Write the appropriate formula. Substitute. |
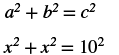 |
| Step 5. Solve the equation. Isolate the variable. Use the definition of the square root. Simplify. Approximate to the nearest tenth. |
 |
Step 6. Check: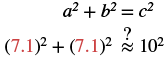 Yes. |
|
| Step 7. Answer the question. | Kelvin should fasten each piece of wood approximately 7.1″ from the corner. |
TRY IT 9.1
John puts the base of a ![]() ladder
ladder ![]() feet from the wall of his house. How far up the wall does the ladder reach?
feet from the wall of his house. How far up the wall does the ladder reach?

Show answer
12 feet
TRY IT 9.2
Randy wants to attach a ![]() string of lights to the top of the
string of lights to the top of the ![]() mast of his sailboat. How far from the base of the mast should he attach the end of the light string?
mast of his sailboat. How far from the base of the mast should he attach the end of the light string?
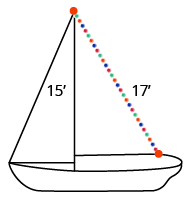
Show answer
8 feet
Summary
 Sum of the Measures of the Angles of a Triangle
Sum of the Measures of the Angles of a Triangle
- For any
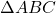 , the sum of the measures is 180°
, the sum of the measures is 180° 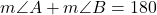
- For any
 Right Triangle
Right Triangle
- A right triangle is a triangle that has one 90° angle, which is often marked with a ⦜ symbol.
- Properties of Similar Triangles
- If two triangles are similar, then their corresponding angle measures are equal and their corresponding side lengths have the same ratio.
Attributions
This chapter has been adapted from “Use Properties of Angles, Triangles, and the Pythagorean Theorem” in Prealgebra (OpenStax) by Lynn Marecek, MaryAnne Anthony-Smith, and Andrea Honeycutt Mathis, which is under a CC BY 4.0 Licence. Adapted by Izabela Mazur. See the Copyright page for more information.

